Tag Archives: Rossby waves

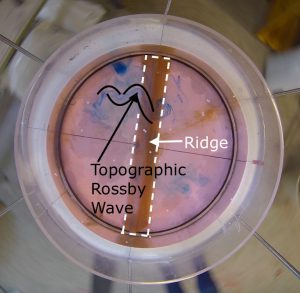
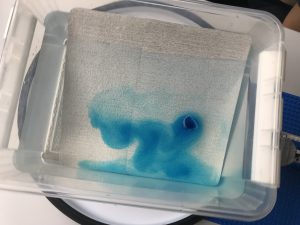
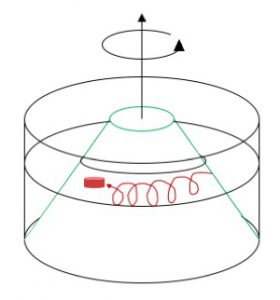
Rossby-#WaveWatchingWednesday

2 Replies

Several of my friends were planning on teaching with DIYnamics rotating tables right now. Unfortunately, that’s currently impossible. Fortunately, though, I have one at home and enjoy playing with it enough that I’m

  1. Playing with it
  2. Making videos of me playing with it
  3. Putting the videos on the internet
  4. Going to do video calls with my friends’ classes, so that the students can at least “remote control” the hands-on experiments they were supposed to be doing themselves.

Here is me introducing the setup:

Today, I want to share a video I filmed on planetary Rossby waves. To be clear: This is not a polished, stand-alone teaching video. It’s me rambling while playing. It’s supposed to give students an initial idea of an experiment we’ll be doing together during a video call, and that they’ll be discussing in much more depth in class. It’s also meant to prepare them for more “polished” videos, which are sometimes so polished that it’s hard to actually see what’s going on. If everything looks too perfect it almost looks artificial, know what I mean? Anyway, this is as authentic as it gets, me playing in my kitchen. Welcome! :-)

In the video, I am using an ice cube, melting on a sloping bottom in a rotating tank, to create planetary Rossby waves. Follow along with the whole process:

Also check out the video below that shows both a top- and side view of a planetary Rossby wave, both filmed with co-rotating cameras.

Previous blog posts with more movies for example here.

Now. What are you curious about? What would you like to try? What would you do differently? Any questions for me? :-)

Rotating tank experiments on a cone

Leave a reply

I had so much fun playing with rotating tank experiments on a cone this afternoon! And with Torge Martin (who I have the awesome #DryTheory2JuicyReality project with) and Rolf Käse (who got me into tank experiments with an amazing lab course back in 2004, that I still fondly remember). We tried so many different things, that I will at some point have to describe in detail, but for now I just need to share the excitement ;-)

Here, for example, a blue fish-shaped ice cube. This experiment is pretty much the topographic Rossby wave experiment described here, except now we aren’t on an inclined plane, but on a cone. Which is basically an infinitely long inclined plane — the ice cube doesn’t encounter a boundary as it travels west, it just goes round and round the tank until it melts. And look at the cool Rossby waves!

Then we did another one of our favourite experiments, the Hadley cell circulation. What was really fascinating to observe was how turbulence the turbulence that was introduced by dripping dye into the tank changed scales. At first, we had the typical 3D pattern with plumes shooting down. But over time, the pattern became more and more organized, larger, and 2D. See below: The blue dye had been in the tank for a little longer than the red dye, so the structures look completely different. But interesting to keep that in mind when interpreting structures we observe!

Here is another view of the same experiment. Since we are cooling in the middle and rotating very slowly (about 3 rotations per minute), the eddy structures aren’t completely 2D, but they are influenced by an overturning component.

This looks even cooler when done on a cone. Can you see how there is both an overturning component (i.e. the plumes running down the slope) and then still a strong column in the middle?

This just looks so incredibly beautiful!

And one last look on the eddies that develop. We saw that there are cyclonic eddies happening in the center of the tank and anti-cyclonic eddies at the edge. Since we are on a cone, I could imagine that it’s just due to conservation of vorticity. Stuff that develops near the center and moves down the slope needs to spin cyclonically since the columns are being stretched, and on the other hand things that develop near the edge must move up the slope, thus columns being compressed. What do you think? What would be your explanation?

Topographic Rossby waves in a tank

Leave a reply

This experiment just doesn’t want to be filmed by me. Even though I spent more time on preparation of this experiment than on almost any other experiment I have ever done! I have written up the theory behind this experiment, run it with a blob of dye to visualize the wave, then with a ring of dye. But for some reason, something goes wrong every time. Like people opening the door to the lab to come and visit me just the very second I am about to put dye into the tank, resulting in me jumping and a lot of dye ending up in the wrong spots… Or the tank itself getting the hickups. Or the cameras not playing nicely if for once the experiment itself goes well.

Anyway, it is still a very cool experiment! So here are some pictures.

In all those pictures, the tank is rotating a lot more slowly than recommended in the instructions. I thought that might make it all easier to run (5rpm; dial at approximately 7 for GFI big tank, similar to Taylor column). And it looks just fine, except that the restoring force back to the middle isn’t really there (as was to be expected, since the surface is almost flat and the parabolic shape is needed for a difference in water depth).

Third attempt

Below, you see the “ridge”, a piece of hose that connects a solid cylinder in the middle of the tank to the tank’s outer wall. The tank is turning counter-clockwise.

The flow looks substantially different upstream and downstream of the ridge: Upstream, it is laminar and close to the middle cylinder. Downstream, it’s meandering (the Rossby waves!) and diffusive.

Fifth attempt (same as above)

In this experiment, the difference between the flow up- and downstream of the ridge are even more obvious. Look at those eddies!

It’s quite amazing to see how a small disturbance can make the entire system unstable.

 

Topographic Rossby wave

Leave a reply

Next attempt at the topographic Rossby wave! This time with following the geosci.uchicago.edu instructions more closely…

…and then the tank had hickups, so we did get waves, but a lot more diffusive than we had hoped, because the tank slowed down a lot more and in a more bumpy fashion than I had planned…

Setup of the topographic Rossby wave experiment

For a demonstration of topographic Rossby waves, we want the Coriolis parameter f to stay constant but have the depth H change. We use the instructions by geosci.uchicago.edu as inspiration for our experiment and

  • build a shallow ridge into the tank, from a cylinder in the middle to the outer wall. My solution: Take a 1.5 cm (outer) diameter hose, tape it to the bottom of a tank to achieve a ridge with smooth edges
  • 7 cm water depth
  • spin up the tank to approximately 26 rpm
  • wait for it to reach solid body rotation (ca 10 min)
  • introduce dye all around the cylinder in the middle
  • reduce rotation slightly, to approximately 23 rpm so the water inside the tank moves relative to the tank itself, and thus has to cross the ridge which is fixed to the tank
  • watch it change from laminar flow to eddies downstream of the ridge. Hopefully ;-)

Planetary Rossby waves

Leave a reply

I ran my new favourite experiment again, the planetary Rossby waves. They work super well on the DIYnamics table we built in Kiel and they also worked really well the other day in Bergen.

I mainly ran it today because I wanted to get an idea of how robust the experiment is, i.e. what to prepare for when running it with students in terms of weird results that might have to be explained.

Here is a side view of the square tank with a sloping bottom. The blue ice cube is melting. The melt water is forming a Taylor column down to the bottom of the tank. Some of it then continues down the slope.

Here we are looking at the slope and see the same thing (plus the reflection at the surface). Note how the ice cube and its  meltwater column have already moved quite a bit from the corner where I released it!

When the blue ice cube had crossed half the width of the tank and the blue melt water had almost reached the other edge, I released a green ice cube. Sadly the dye wasn’t as intense as the blue one. But it’s quite nice that the wave length between the individual plumes going down the slope stays the same, for all the blue plumes as well as for the new green ones.

Here in the side view we see the columns of the blue and green ice cube, and we also see that each of the plumes going down the slope still has Taylor columns attached at its head.

Here is an accelerated movie of the experiment, 20x faster than real time. Not sure why there is still sloshing in the tank (this time I made sure it was level), but it’s very nice to see that the ice cubes are spinning cyclonically, faster than the tank! As they should, since they are sitting on Taylor columns…

I think next time I really want to make a side view movie of the Taylor columns and plumes. Not quite sure yet how I will manage the lights so they don’t get super annoying…

Topographic Rossby wave

Leave a reply

Finally trying the topographic Rossby wave experiment I wrote about theoretically here!

And it is working — ok-ish. If you know what you are looking for, you can kind of see it. So check out the picture above so you know what you expect to see below ;-) We are rotating the tank fairly rapidly (and there are a lot of inertial oscillations in the water even after a long spinup, don’t know why) and then slow it down just a little bit to create a current relative to the topography.

So it turns out that following instructions better might actually have been a good idea. We will do that some other day on a different rotating table.

Here is what we did today:

Setup of the topographic Rossby wave experiment

For a demonstration of topographic Rossby waves, we want the Coriolis parameter f to stay constant but have the depth H change. We use the instructions by geosci.uchicago.edu as inspiration for our experiment and

  • build a shallow ridge into the tank. My solution: Take a 2.3 cm (outer) diameter hose, tape it to the bottom of a tank to achieve a ridge with smooth edges
  • important difference to the geosci.uchicago.edu setup: We are just using our cylindrical tank without a solid cylinder in the middle. Therefore our ridge goes all the way across the tank. Main reason is that our rotating tank’s camera sits on six rods, so at fast rotations it is very difficult to insert dye and I thought this way might be easier. But that might not actually be true…
  • 10 cm water depth
  • spin up the tank to approximately 26 rpm (23 seconds for 10 rotations == 36.5 on the display of GFI’s large rotating table)
  • wait for it to reach solid body rotation (ca 10 min)
  • introduce dye upstream of the ridge,
  • reduce rotation slightly, to approximately 23 rpm (26 seconds for 10 rotations == 33 on the display of GFI’s large rotating table) so the water inside the tank moves relative to the tank itself, and thus has to cross the ridge which is fixed to the tank
  • watch it change from laminar flow to eddies downstream of the ridge. Hopefully ;-)

Planetary Rossby waves on Beta-plane. A super easy tank experiment!

3 Replies

This is seriously one of the easiest tank experiments I have ever run! And I have been completely overthinking it for the last couple of weeks.

Quick reminder: This is what we think hope will happen: On a slope, melt water from a dyed ice cube will sink, creating a Taylor column that will be driven down the slope by gravity and back up the slope by vorticity conservation, leading to a “westward” movement in a stretched, cyclonic trajectory.

We are using the DIYnamics setup: A LEGO-driven Lazy Susan. And as a tank, we are using a transparent plastic storage box that I have had for many years, and the sloping bottom is made out of two breakfast boards that happened to be a good size.

Water is filled to “just below the edge of the white clips when they are in the lower position” (forgot to take measurements, this is seriously what I wrote down in my notes. We didn’t really think this experiment would work…)

The tank is then rotated at the LEGO motor’s speed (one rotation approximately every 3 seconds) and spun into solid body rotation. We waited for approximately 10 minutes, although I think we had reached solid body rotation a lot faster. But we had a lot of surface waves that were induced by some rotation that we couldn’t track down and fix. But in the end they turned out to not matter.

To start the experiment, Torge released a blue ice cube in the eastern corner of the shallow end. As the ice cube started melting, the cold melt water sank down towards the ground, where it started flowing towards the bottom of the tank. That increased the water column’s positive relative vorticity, which drove it back up the slope.

This was super cool to watch, especially since the ice cube started spinning cyclonically itself, too, so was moving in the same direction and faster than the rotating tank.

You see this rotation quite well in the movie below (if you manage to watch without getting seasick. We have a co-rotating setup coming up, it’s just not ready yet…)

Very soon, these amazing meandering structures appear: Rossby waves! :-)

And over time it becomes clear that the eddies that are being shed from the column rotating with the ice cubes are constant throughout the whole water depth.

It is a little difficult to observe that the structure is really the same throughout the whole water column since the color in the eddies that were shed is very faint, especially compared to the ice cube and the melt water, but below you might be able to spot it for the big eddy on the left.

Or maybe here? (And note the surface waves that become visible in the reflection of the joint between the two breakfast boards that make up the sloping bottom. Why is there so much vibration in the system???)

You can definitely see the surface-to-bottom structures in the following movie if you don’t let yourself be distracted by a little #HamburgLove on the back of the breakfast boards. Watching this makes you feel really dizzy, and we’ve been starting at this for more than the 8 seconds of the clip below ;-)

After a while, the Taylor column with the ice cube floating on top starts visibly moving towards the west, too. See how it has almost reached the edge of the first breakfast board already?

And because this was so cool, we obviously had to repeat the experiment. New water, new ice cube.

But: This time with an audience of excited oceanographers :-)

This time round, we also added a second ice cube after the first one had moved almost all the way towards the west (btw, do you see how that one has this really cool eddy around it, whereas the one in the east is only just starting to rotate and create its own Taylor column?)

And last not least: Happy selfie because I realized that there are way too few pictures like this on my blog, where you see what things look like (in this case in the GEOMAR seminar room) and who I am playing with (left to right: Torge, Franzi, Joke, Jan) :-)

Rossby waves in a rotating tank — three different demonstrations

3 Replies

For both of my tank experiment projects, in Bergen and in Kiel, we want to develop a Rossby wave demonstration. So here are my notes on three setups we are considering, but before actually having tried any of the experiments.

Background on Rossby waves

I recently showed that rotating fluids behave fundamentally differently from non-rotating ones, in that they mainly occur in the horizontal and thus are “only” 2 dimensional. This works really well as long as several conditions are met, namely the water depth can’t change, nor can the rotation of the fluid. But this is not always the case, so when either the water depth or the rotation does change, the flow still tries to conserve potential vorticity and stay 2 dimensional, but now displays so-called Rossby waves.

Here are different setups for Rossby wave demonstrations I am currently considering.

Topographic Rossby wave

For a demonstration of topographic Rossby waves, we want the Coriolis parameter f to stay constant but have the depth H change. We use the instructions by geosci.uchicago.edu as inspiration for our experiment and

  • build a shallow ridge into the tank. They use an annulus and introduce the ridge at a random longitude, we could also build one across the center of the tank all the way to both sides to avoid weird things happening in the middle (or introduce a cylinder in the middle to mimic their annulus)
  • spin up the tank to approximately 26 rpm (that seems very fast! But that’s probably needed in order to create a parabolic surface with large height differences)
  • wait for it to reach solid body rotation (ca 10 min)
  • reduce rotation slightly, to approximately 23 rpm so the water inside the tank moves relative to the tank itself, and thus has to cross the ridge which is fixed to the tank
  • introduce dye upstream of the ridge, watch it change from laminar flow to eddies downstream of the ridge (they introduced dye at the inner wall of their annulus when the water was in solid body rotation, before slowing down the tank).

What are we expecting to see?

In case A, we assume that the rotation of the tank is slow enough that the surface is more or less flat. This will certainly not be the case if we rotate at 26rpm, but let’s discuss this case first, anyway. If we inject dye upstream of the obstacle, the dye will show that the current is being deflected as it crosses the ridge, to one direction as the water columns are getting shorter as they move up the ridge, then to the other direction when the columns are stretched going down the obstacle again. Afterwards, since the water depth stays constant, they would just resume a circular path.

In case B, however, we assume a parabolic surface of the tank, which we will have for any kind of fast-ish rotation. Initially, the current will move similarly to case A. But once it leaves the ridge, if it has any momentum in radial direction at all, it will overshoot its circular path, moving into water with a different depth. This will then again expand or compress the columns, inducing relative vorticity, leading to a meandering current and eddies downstream of the obstacle (probably a lot more chaotic than drawn in my sketch).

So in both cases we initially force the Rossby wave by topography at the bottom of the tank, but then in case B we sustain it by the changes in water depth due to the sloping surface.

My assessment before actually having run the experiment: The ridge seems fairly easy to construct and the experiment easy enough to run. However what I am worried about is the change in rotation rate and the turbulence and Ekman layers that it will introduce. After all, slowing down the tank is what we do create both turbulence and Ekman layers in demonstrations, and then we don’t even have an obstacle stuck in the tank. The instructions suggest a very slight reduction in rotation, so we’ll see how that goes…

Planetary Rossby waves on beta-plane

If we want to have more dramatic changes in water depth H than relying on the parabolic shape of the surface, another option is to use a rectangular tank and insert a sloping bottom as suggested by the Weather in a Tank group here. We are now operating on a Beta plane with the Coriolis parameter f being the sum of the tank’s rotation and the slope of the bottom.

Following the Weather in a Tank instructions, we plan to

  • fill a tank with a sloping bottom (slope approximately 0.5)
  • spin it at approximately 15 rpm until it reaches solid body rotation (15-20 minutes later)
  • place a dyed ice cube (diameter approximately 5 cm) in the north-eastern corner of the tank

What do we expect to see?

Ice cube and its trajectory (in red) on a sloping bottom in a rotating tank. Note: This sketch does not include the melt water water column!

Above is a simplified sketch of what will (hopefully!) happen. As the ice cube starts melting, melt water is going to sink down towards the sloping bottom, stretching the water column. This induces positive relative vorticity, making the water column spin cyclonically. As the meltwater reaches the sloping bottom, it will flow downhill, further stretching the water column. This induces more positive relative vorticity still, so the water column, and with it the ice cube, will start moving back up the slope until they reach the “latitude” at which the ice cube initially started. Having moved up the slope into shallower water, the additional positive vorticity induced by the stretching as the water was flowing down the slope has now been lost again, so rather than spinning cyclonically in one spot, the trajectory is an extended cycloid.

My assessment here (before having run it): I find this experiment a little more unintuitive because there are the different components of stretching contributing to the changes in relative vorticity. And from the videos I’ve seen, we don’t really get a clear column moving, but there are cyclonic eddies in the boundary layer that are shed. So I think this might be more difficult to observe and interpret. But I am excited to try!

Planetary Rossby wave on a cone (cyclical beta-plane?)

Following the Weather in a Tank instructions, we plan to also do the experiment as above but with cyclical boundary conditions, by using a cone in a cylindrical tank instead of a sloping bottom in a rectangular one.

The experiment is run in the same way as the one above (except they suggest a slightly slower rotation of 10 rpm). Physics are the same as before, except that now the transfer to reality should be a little easier, since we now have Rossby waves that can really run all the way around the pole. Also the experiment can be run for a longer time, since we don’t run into a boundary in the west if we are moving around and around the pole.

Ice cube and its trajectory (in red) on a cone in a rotating tank. Note: This sketch does not include the melt water column!

My assessment before actually having run the experiment: This shouldn’t be any more difficult to run, observe or interpret than the one above (at least once we’ve gotten our hands on a cone). Definitely want to try this!

Vorticity and Rossby waves

1 Reply

Usually when we talk about waves on this blog, we talk about surface- or sometimes internal waves, but my waves almost always oscillate vertically. Today, we’ll mix things up a little: Rossby waves are waves in the horizontal plane. They exist for example as oscillations on the atmosphere’s jet stream. In order to understand what causes them, we need the concept of vorticity, which I will go over first before giving examples for Rossby waves.

Vorticity

Vorticity is a measure of how much a fluid is rotating. Generally speaking, once a fluid is in rotation, it wants to keep rotating (as we saw for example with the bottom Ekman layers in a rotating tank, where the water inside kept on rotating after the tank was stopped, until it was slowed down by friction). There are several components that are at play here — the rotation that we see when looking at eddies, the rotation of the Earth, and others — which I will go over in the following.

Relative vorticity

The easiest way to imagine what “vorticity” is (and the only one that I’ve talked about on this blog, see here), is to think of a little float in a flow. In a vorticity-free flow, that little float will always keep its orientation (see below). However if there is shear in the flow, i.e. the flow field carries vorticity, it will start to turn.

vorticity1

Flow fields without vorticity (top) and with vorticity (bottom). 

Relative vorticity is what we see for example when looking al leaves swirling in rivers.

Planetary vorticity

Since the Earth is turning (and all the water on it with it), the water also carries planetary vorticity, i.e. the rotation of the Earth, which is the Coriolis parameter. The Coriolis parameter is largest at the poles and zero at the equator, meaning the rotation changes with latitude.

The rotation of the Earth is clearly important enough for us to want to spin our tanks to simulate its effects on ocean currents.

Absolute vorticity

The sum of relative and planetary vorticity is called absolute vorticity: This is how much any fluid column is rotating in total, including all possible components of rotation (which are only the two mentioned above, but still…).

Potential vorticity

One more factor that can influence the rotation of a fluid parcel is the water depth. When water depth increases, columns of water get expanded vertically (since, for continuity reasons, they still have to go all the way from surface to bottom, even if the distance is now larger) or, if water depth decreases, squished. Similarly to figure skaters that stretch or crouch to increase or decrease their rotation, the expansion of a column of water leads to a change in its rotation.

Potential vorticity is defined as absolute vorticity over water depth.

Conservation of potential vorticity leads to waves

Potential vorticity is conserved, so if water depth, planetary vorticity changes or relative vorticity changes, something else has to change to compensate. And if water changes how much it is rotating, this leads to meanders in currents, i.e. waves.

Depth is constant, but latitude changes: Planetary Rossby waves

Planetary vorticity changes with latitude, therefore if a water parcel moves in north-south direction over constant water depth, its relative vorticity needs to change in order for potential vorticity to be conserved. This leads to so-called planetary Rossby waves, where currents in the ocean or the atmosphere start oscillating in north-south direction (see figure below).

At position 1, a fluid parcel gets for any random reason pushed northward. As it moves north, its planetary vorticity increases and its relative vorticity therefore has to decrease to compensate (2). This leads to southward movement, but the initial latitude (3) is overshot a little (4). This again leads to a change in relative vorticity (4), which brings the water parcel back to its initial latitude (5), but it overshoots again… So this mechanism leads to a wave-like motion in the horizontal plane, with the phase of the wave propagating westward.

This can happen at any latitude, even at the equator where “equatorial planetary Rossby waves” occur. At the equator f=0, but as soon as the water column has moved slightly north or south from the equator, f kicks in and drives the water column back to the equator (where it then overshoots, is turned back, overshoots again…….).

Latitude is constant but depth changes: Topographic waves

If a current encountered a ridge, the water depth changes and the current thus gets deflected. This motion is called topographic wave: When a water column gets stretched, it gains relative vorticity, making it rotate cyclonically. When it runs into shallower water, it loses relative vorticity and starts turning the other way.

I’m hoping to set up demonstrations for both types of Rossby waves soon. Stay tuned! :-)